Researchers Solve the Mystery of Wrinkling Patterns and Fingerprints

Ever wondered why our fingers have these patterns on them, almost geometric – some round, some boat shaped? of course, that does aid us in better gripping and holding but what are the chances? What are the chances that our fingers are ridged the way they are?
Alright. Let’s talk about it in this way. Have you ever wondered why a grape wrinkles the way it does, into a raisin? Statistically speaking, what is the probability that this very grape wrinkles with these very patterns into a raisin? Going further ahead, could there be a mathematical function that explains patterns on curved surfaces?
Now a team of MIT mathematicians and engineers has developed a mathematical theory, confirmed through experiments, that predicts how wrinkles on curved surfaces take shape. From their calculations, they determined that one main parameter—curvature—rules the type of pattern that forms: The more curved a surface is, the more its surface patterns resemble a crystal-like lattice.
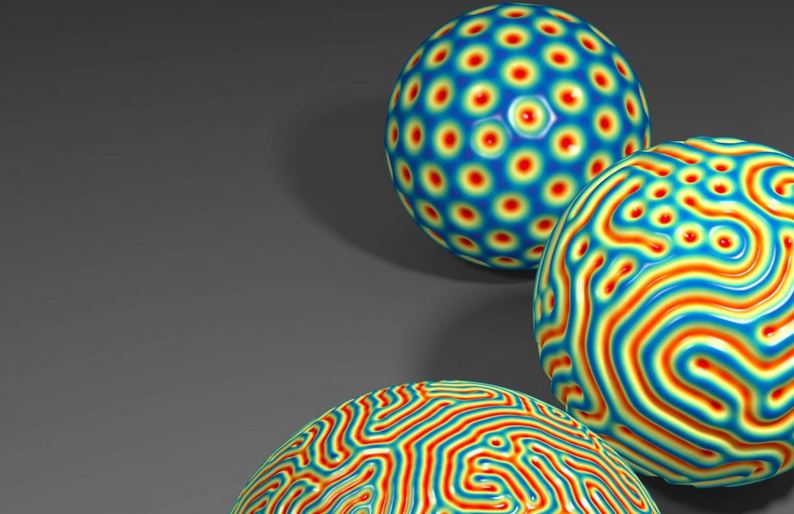
In past experiments, ping-pong sized balls were manufactured in order to investigate how their surface patterns may affect the sphere’s drag. A characteristic transition in the sphere’s surface patterns was observed as air was slowly sucked out: As the sphere’s surface became compressed, it began to dimple, forming a pattern of regular hexagons before giving way to a more convoluted, labyrinthine configuration, similar to fingerprints.

Also See: Physicists Create Mobius Strips by Polarization of Light
The team predicted that there exists a mathematical framework for describing wrinkling, in the form of elasticity theory. Combining ideas from fluid mechanics with elasticity theory, a simplified equation was derived that accurately predicts wrinkling pattern.
In computer simulations, the researchers confirmed that their equation was indeed able to reproduce correctly the surface patterns observed in experiments. They were therefore also able to identify the main parameters that govern surface patterning.
As it turns out, curvature is one major determinant of whether a wrinkling surface becomes covered in hexagons or a more labyrinthine pattern: The more curved an object, the more regular its wrinkled surface. The thickness of an object’s shell also plays a role: If the outer layer is very thin compared to its curvature, an object’s surface will likely be convoluted, similar to a fingerprint. If the shell is a bit thicker, the surface will form a more hexagonal pattern.
Stay tuned for trending and latest science and technology news from fossBytes.